-
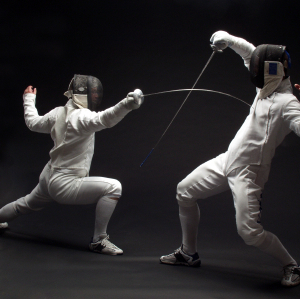
Four sporty brainteasers in the context of fencing, hockey, football and international medal tables. This activity challenges students to be resourceful, to think logically and to work systematically, and is designed to be accessible to secondary maths students at Key Stages 3 and 4.
-

Usain Bolt, the "fastest man on the planet", aims to get his 100 metre world record of 9.58 seconds down to 9.40 seconds. But is there an ultimate limit which no runner can possibly break? In this article, aimed at the general public and older students, Tony Crilly looks at whether mathematics can give us the answer.
-

The Olympic and Paralympic Games are a global celebration of excellence, determination and effort. Much of the media coverage, though, tends to focus on each country's tally of gold medals. This article by Rob Eastaway and John Haigh explores some of the mathematical questions raised by Olympic success. How well did Britain really do in 2008 in Beijing, with nineteen golds? Are some gold medals worth more than others? And are there even some sports the Olympics should drop?
-

Can you work out which order these thirteen nations finished in after competing? This activity presents an exercise in strategic thinking, accessible to lower secondary students (but hinting at the more advanced mathematics of sorting algorithms that they might meet if continuing to study maths at A-level). It is aimed at Key Stage 3 students.
-

Can you use data from the 2012 London Olympics medal tables to decide which country has the most naturally athletic population? This data-handling activity encourages mathematical investigation and discussion and is designed to be accessible to secondary maths students at Key Stages 3 and 4.
-

This activity suggests a number of investigative projects, focusing on athletics and swimming, exploring some of the trends between performances in different sports at the same time in history and in the rate of improvement of records over time. These project ideas provide opportunities for mathematical modelling and for handling, processing and analysing data, and are aimed at GCSE and A Level students (Key Stages 4 and 5).
-

After every Olympics, there is speculation about which country performed best. Should we really be surprised when China, with its huge population, and the US, with its combination of high GDP and population, top the medal table? Can we take a look at the medal tables and see which countries did indeed perform better than expected?
-
In the 2008 Beijing Olympics the UK finished 4th in the total medal count. How might we do in 2012, and does the country hosting the Olympics have an edge?

